A formal operator involving Fermatian numbers

Carlos M. da Fonseca and Anthony G. Shannon
Notes on Number Theory and Discrete Mathematics
Print ISSN 1310–5132, Online ISSN 2367–8275
Volume 30, 2024, Number 3, Pages 491–498
DOI: 10.7546/nntdm.2024.30.3.491-498
Full paper (PDF, 200 Kb)

Details

Authors and affiliations

Carlos M. da Fonseca
1 Kuwait College of Science and Technology
Doha District, Safat 13133, Kuwait
2 Chair of Computational Mathematics, University of Deusto
48007 Bilbao, Spain

Anthony G. Shannon
3 Honorary Fellow, Warrane College, University of New South Wales
2033, Australia

Abstract

In this note, old and new properties of Fermatian numbers \underline{z}_n= \dfrac{1-z^n}{1-z} are recalled. A new formal operator is defined and some identities and extensions are discussed.

Keywords

  • Fermatian numbers
  • Recurrence relation
  • Formal operators

2020 Mathematics Subject Classification

  • 11B39
  • 11B75
  • 11B65
  • 05A30

References

  1. Anđelic, M., da Fonseca, C. M., & Yılmaz, F. (2022). The bi-periodic Horadam sequence and some perturbed tridiagonal 2-Toeplitz matrices: A unified approach. Heliyon, 8, Article E08863.
  2. Bondarenko, B. A. (1993). Generalized Pascal Triangles and Pyramids: Their Fractals, Graphs and Applications. Translated by R. C. Bollinger. Santa Clara, CA: The Fibonacci Association.
  3. Carlitz, L. (1940). A set of polynomials. Duke Mathematical Journal, 6, 486–504.
  4. Carlitz, L. (1948). q-Bernoulli numbers and polynomials. Duke Mathematical Journal, 15, 987–1000.
  5. Cox, N. J., & Hoggatt Jr., V. E. (1970). Some universal counter examples. The Fibonacci Quarterly, 8, 242–248.
  6. Guy, R. K. (1994). Unsolved Problems in Number Theory. (2nd ed.). New York, Springer-Verlag, pp. 28–29.
  7. Hoggatt Jr., V. E., & Bicknell, M. (1969). Diagonal sums of generalized Pascal triangles. The Fibonacci Quarterly, 7, 341–358.
  8. Jameson, G. J. O. (2011). Finding Carmichael numbers. Mathematical Gazette, 95, 244–255.
  9. Pinch, R. G. E. (2000). The pseudoprimes up to 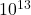10^{13}. Algorithmic Number Theory, Lecture Notes in Computer Science, Vol. 1838, 459–473. Springer, Leiden.
  10. Pomerance, C., Selfridge, J. L., & Wagstaff Jr., S. S. (1980). The pseudoprimes to 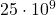25 \cdot 10^9. Mathematics of Computation, 35, 1003–1026.
  11. Sàndor, J., & Atanassov, K. T. (2021). Chapter 1. Arithmetic Functions. New York, Nova Science Publishers.
  12. Shanks, D. (1993). Solved and Unsolved Problems in Number Theory (4th ed.). New York, Chelsea, pp. 115-117.
  13. Shannon, A. G. (2003). Some Fermatian special functions. Notes on Number Theory and Discrete Mathematics, 9(4), 73–82.
  14. Shannon, A. G. (2004). Some properties of Fermatian numbers. Notes on Number Theory and Discrete Mathematics, 10(2), 25–33.
  15. Shannon, A. G. (2004). A Fermatian Staudt–Clausen Theorem. Notes on Number Theory and Discrete Mathematics, 10(4), 89–99.
  16. Shannon, A. G. (2010). Fermatian analogues of Gould’s generalized Bernoulli polynomials. Notes on Number Theory and Discrete Mathematics, 16(4), 14–17.
  17. Shannon, A. G. (2019). Applications of Mollie Horadam’s generalized integers to Fermatian and Fibonacci numbers. Notes on Number Theory and Discrete Mathematics, 25(2), 113–126.
  18. Sloane, N. J. A. (2024). The On-Line Encyclopedia of Integer Sequences. Available online at: http://oeis.org.
  19. Sylvester, J. J. (1912). Chapters 1, 4. The Collected Papers, Volume IV (1882–1897). Cambridge University Press.
  20. Udrea, G. (1996). A note on the sequence (W_n)_{n \ge 0} of A. F. Horadam. Portugaliae Mathematica, 53, 143–155.
  21. Vandiver, H. S. (1941). Simple explicit expressions for generalized Bernoulli numbers. Duke Mathematical Journal, 8, 575–584.
  22. Vorob’ev, N. N. (1980). Criteria for Divisibility. University of Chicago Press, Chapter 3.
  23. Ward, M. (1936). A calculus of sequences. American Journal of Mathematics, 58, 255–266.
  24. Weisstein, E. W. (2005). Poulet Number. MathWorld – A Wolfram Web Resource. Available online at: https://mathworld.wolfram.com/PouletNumber.html

Manuscript history

  • Received: 9 April 2024
  • Revised: 16 August 2024
  • Accepted: 18 August 2024
  • Online First: 26 September 2024

Copyright information

Ⓒ 2024 by the Authors.
This is an Open Access paper distributed under the terms and conditions of the Creative Commons Attribution 4.0 International License (CC BY 4.0).

Related papers

  1. Shannon, A. G. (2003). Some Fermatian special functions. Notes on Number Theory and Discrete Mathematics, 9(4), 73–82.
  2. Shannon, A. G. (2004). Some properties of Fermatian numbers. Notes on Number Theory and Discrete Mathematics, 10(2), 25–33.
  3. Shannon, A. G. (2004). A Fermatian Staudt–Clausen Theorem. Notes on Number Theory and Discrete Mathematics, 10(4), 89–99.
  4. Shannon, A. G. (2010). Fermatian analogues of Gould’s generalized Bernoulli polynomials. Notes on Number Theory and Discrete Mathematics, 16(4), 14–17.
  5. Shannon, A. G. (2019). Applications of Mollie Horadam’s generalized integers to Fermatian and Fibonacci numbers. Notes on Number Theory and Discrete Mathematics, 25(2), 113–126.

Cite this paper

Da Fonseca, C. M., & Shannon, A. G. (2024). A formal operator involving Fermatian numbers. Notes on Number Theory and Discrete Mathematics, 30(3), 491-498, DOI: 10.7546/nntdm.2024.30.3.491-498.

Comments are closed.