[go: up one dir, main page]
Jump to content

Ellipse

Edit links
From Wikiquote
An ellipse

In mathematics, an ellipse is a curve in a plane surrounding two focal points such that the sum of the distances to the two focal points is constant for every point on the curve. As such, it is a generalization of a circle, which is a special type of an ellipse having both focal points at the same location. The shape of an ellipse (how "elongated" it is) is represented by its eccentricity, which for an ellipse can be any number from 0 (the limiting case of a circle) to arbitrarily close to but less than 1.

Quotes

[edit]
  • Two years before the publication of his Dioptrics, viz. in 1609, Kepler had given to the world his great work entitled "The New Astronomy, or Commentaries on the Motions of Mars." The discoveries which this volume records form the basis of physical astronomy. The inquiries by which he was led to them began in that memorable year 1601, when he became the colleague or assistant of Tycho. ...Having tried in vain to represent the motion of Mars by an uniform motion in a circular orbit, and by the cycles and epicycles with which Copernicus had endeavoured to explain the planetary inequalities, Kepler was led, after many fruitless speculations, to suppose the orbit of the planet to be oval; and from his knowledge of the conic sections, he afterward determined it to be an ellipse, with the sun placed in one of its foci. He then ascertained the dimensions of the orbit; and, by a comparison of the times employed by the planet to complete a whole revolution or any part of one, he discovered that the time in which Mars describes any arches of his elliptic orbit, were always to one another as the areas contained by lines drawn from the focus, or the centre of the sun, to the extremities of the respective arches; or, in other words, that the radius vector, or the line joining the Sun and Mars described equal areas in equal times. By examining the inequalities of the other planets he found that they all moved in elliptic orbits, and that the radius vector of each described areas proportional to the times. These two great results are known by the name of the first and second laws of Kepler. The third law, or that which relates to the connexion between the periodic times and the distances of the planets, was not discovered till a later period of his life.
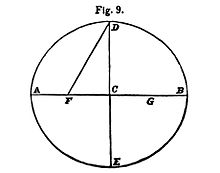
Ellipse in Thomas Dick's "Celestial Scenery; or, the Wonders of the Planetary System Displayed," Fig.9
  • The figure A D B E [in Figure 9] represents the form of a planetary orbit, which is that of an oval or ellipse. The longest diameter is A B; the shorter diameter D E. The two points F and G are called the foci of the ellipse, around which, as two central points, the ellipse is formed. The sun is not placed in C, the center of the orbit, but at F, one of the foci of the ellipse. When the planet, therefore, is at A it is nearest the sun, and is said to be in its perihelion; its distance from the sun gradually increases until it reaches the point, B, when it is at its greatest distance the sun, and is said to be in its aphelion; when it arrives at the points D and E of its orbit, it said to be at the mean distance. ...F D , the mean distance of the planet from the sun... The orbits of some of the planets are more elliptical than others. ...Most of the orbits, except those of some of the new planets, approach very nearly to the circular form.
    • Thomas Dick, Celestial Scenery; or, the Wonders of the Planetary System Displayed; Illustrating the Perfections of Deity and a Plurality of Worlds in The Complete Works of Thomas Dick, LL.D.: Eleven Volumes in Two, Vol. 2 (1873)
  • Lagrange, struck with the circumstance that the calculus had never given any inequalities but such as were periodical, applied himself to the investigation of a general question, from which he found by a method peculiar to himself and independent of any approximation, that the inequalities produced by the mutual action of the planets must in effect be all periodical; that the periodical changes are confined within narrow limits; that none of the planets ever has been or ever can be a comet moving in a very eccentric orbit; but that the planetary system oscillates as it were round a medium state from which it never deviates far: that amid all the changes which arise from the mutual actions of the planets, two things remain perpetually the same, viz. the length of the greater axis of the ellipse which the planet describes, and its periodical time round the sun; or, which is the same thing, the mean distance of each planet from the sun and its mean motion remain constant. The plane of the orbit varies, the species of the ellipse and its eccentricity change, but never, by any means whatever, the greater axis of the ellipse, or the time of the entire revolution of the planet. The discovery of this great principle, which we may consider as the bulwark that secures the stability of our system, and excludes all access to confusion and disorder, must render the name of Lagrange for ever memorable in science, and ever revered by those who delight in the contemplation of whatever is excellent and sublime. After Newton's discovery of the elliptic orbits of the planets from gravitation, Langrange's discovery of their periodical inequalities is, without doubt, the noblest truth in physical astronomy, and in respect of the doctrine of final causes, it may truly be regarded as the greatest of all.
  • The planetary orbits are not exact circles, but are more or less elliptical, the Sun being situated not at the centre, but at one of the foci of the ellipse. The distance of either focus from the centre indicates the eccentricity of the ellipse. On account of this eccentricity the distance of a planet from the Sun, and its velocity of revolution, vary in different parts of its orbit.
Moon's Orbit in Benjamin Harrow's "From Newton to Einstein" (1920) p.9
  • If the earth attracts the moon, why does not the moon fall to the earth?
    A glance at the accompanying figure will help to answer this question. We must remember that the moon is not stationary, but travelling at tremendous speed—so much so, that it circles the entire earth every month. Now if the earth were absent the path of the moon would be a straight line, say MB, If, however, the earth exerts attraction, the moon would be pulled inward. Instead of following the line MB it would follow the curved path MB'. And again, the moon having arrived at B', is prevented from following the line B'C, but rather B'C'. So that the path instead of being a straight line tends to become curved. From Kepler's researches the probabilities were that this curve would assume the shape of an ellipse rather than a circle.
    ...Kepler's observations of the movements of the planets around the sun was of inestimable value; for from these Newton deduced the hypothesis that attraction varies inversely as the square of the distance. Making use of this hypothesis, Newton calculated what the attractive power possessed by the earth must be in order that the moon may continue in its path. He next compared this force with the force exerted by the earth in pulling the apple to the ground, and found the forces to be identical! "I compared," he writes, "the force necessary to keep the moon in her orb with the force of gravity at the surface of the earth, and found them answer pretty nearly!" One and the same force pulls the moon and pulls the apple—the force of gravity. Further, the hypothesis that the force of gravity varies inversely as the square of the distance had now received experimental confirmation.
    • Benjamin Harrow, From Newton to Einstein: Changing Conceptions of the Universe (1920)
  • Imagine but a single planet revolving about the sun. According to Newton's law of gravitation, the planet's path would be that of an ellipse—that is, oval—and the planet would travel indefinitely along this path. According to Einstein the path would also be elliptical, but before a revolution would be quite completed, the planet would start along a slightly advanced line, forming a new ellipse slightly in advance of the first. The elliptic orbit slowly turns in the direction in which the planet is moving. After many years—centuries—the orbit will be in a different direction. The rapidity of the orbit's change of direction depends on the velocity of the planet. Mercury moving at the rate of 30 miles a second is the fastest among the planets. It has the further advantage over Venus or the earth in that its orbit... is an ellipse, whereas the orbits of Venus and the earth are nearly circular; and how are you going to tell in which direction a circle is pointing?
    Observation tells us that the orbit of Mercury is advancing at the rate of 574 seconds (of arc) per century. We can calculate how much of this is due to the gravitational influence of other planets. It amounts to 532 seconds per century. What of the remaining 42 seconds? ...
    This discrepancy between theory and observation remained one of the great puzzles in astronomy until Einstein cleared up the mystery. According to Einstein's theory the mathematics of the situation is simply this: in one revolution of the planet the orbit will advance by a fraction of a revolution equal to three times the square of the ratio of the velocity of the planet to the velocity of light. When we allow mathematicians to work this out we get the figure 43, which is certainly close enough to 42 to be called identical with it.
    • Benjamin Harrow, From Newton to Einstein: Changing Conceptions of the Universe (1920)
  • According to Newton's law an isolated planet in its motion around a central sun would describe, period after period, the same elliptical orbit; whereas Einstein's laws lead to the prediction that the successive orbits traversed would not be identically the same. Each revolution would start the planet off on an orbit very approximately elliptical, but with the major axis of the ellipse rotated slightly in the plane of the orbit. When calculations were made for the various planets in our solar system, it was found that the only one which was of interest from the standpoint of verification of Einstein's formulas was Mercury.
  • Mercury. The inclination and eccentricity of orbit are greater than those of any of the planets. In obedience to Kepler's law the orbit is an ellipse, one of whose foci is at the sun...
    Venus. The eccentricity of the orbit is so small that in the plot its centre is scarcely distinguishable from the sun. As a consequence the planet revolves at a nearly uniform velocity. ...
    Mars. While the eccentricity of Mars' orbit is less than that of Mercury's, its linear eccentricity or actual distance from its center... to the sun, is greater.
    • Frederic R. Honey, "Morning and Evening Stars for 1917, Scientific American Vol. 116 (1917)
  • Parabola, ellipse, hyperbola, and circle are called conic sections, or simply conics, because they can all be obtained as plane sections of a circular cone. They were originally studied from that point of view, and nearly all their elementary properties that are known to-day were proved by the Greek geometers more than two thousand years ago. The conic sections are of especial interest because of the fact that the paths of all the heavenly bodies are curves of this kind. This fact was first established by the great German astronomer and mathematician Johannes Kepler, in 1609. He showed that the planet Mars moves in an ellipse; the other planets, including of course the earth, do the same, while many comets move in parabolas or hyperbolas.
  • Since the ellipse is the most important of all conic sections in the study of the orbits of the planets, we discuss these curves in more detail, noting that the circle is a special case of an ellipse or, put differently, the ellipse is a symmetrical distortion of a circle.
    • Loyd Motz & Jefferson Hane Weaver, Conquering Mathematics: from Arithmetic to Calculus (1991)
  • Just as the geometric properties of a circle are determined by its radius (a single distance), so are all the geometric properties of an ellipse determined by two distances: its semimajor axis a and its semiminor axis b. We say that the circle has a single geometrical degree of freedom, whereas the ellipse has two such degrees of freedom. ...The larger b is for a given value of a, the rounder the ellipse, and the smaller b is for a given value of a, the flatter the ellipse. If b = a, the ellipse is a circle and if b = 0, the ellipse is a straight line. ...its geometrical properties are a kind of average of the geometrical properties of a circle with radius a and a circle of radius b. ...The arc of the ellipse lies between the circumferences of these two circles; it is inscribed in the larger one and circumscribes the smaller one. ...the ellipse is symmetrical with respect to the y and x axes.
    • Loyd Motz & Jefferson Hane Weaver, Conquering Mathematics: from Arithmetic to Calculus (1991)
  • The Ellipse is the most simple of the Conic Sections, most known, and nearest of Kin to a Circle, and easiest describ'd by the Hand in plano. Though many prefer the Parabola before it, for the Simplicity of the Æquation by which it is express'd. But by this Reason the Parabola ought to be preferr'd before the Circle it self, which it never is. Therefore the reasoning from the Simplicity of the Æquation will not hold. The modern Geometers are too fond of the Speculation of Æquations.
  • How can any prediction of the return of a comet be made? and, supposing such a prediction to be possible, How can it thence be shown that this comet of 1835, is the same with those seen in 1682, in 1607, in 1531, and in 1456?
    Before the first of these questions is answered, the reader must be made acquainted with the nature and properties of a certain curve, called by geometers an ellipse.
    • John W. Parker, "The Cometary System.—The Comet of 1835" The Magazine of Popular Science and Journal of the Useful Arts, (1836) Vol. 1
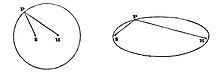
"The Cometary System.—The Comet of 1835"
  • If now the point H be brought nearer to S than it was before, the length of the string remaining the same, and the curve be then described as before, it will be found to have altered its form, so as more nearly to approach that of a circle, as shown in the... figure, and H may be brought so near to S that its form shall scarcely be distinguishable from that of a circle, which figure it would indeed manifestly become, if the point H were made accurately to coincide with S.
    • John W. Parker, "The Cometary System.—The Comet of 1835" The Magazine of Popular Science and Journal of the Useful Arts, (1836) Vol. 1
  • A string, of which both extremities are fixed, passes through a heavy ring. Find the position of equilibrium.
    Let A and B denote the fixed endpoints of the string and X any position of the ring [on the string]. ...The ring must hang as low as possible. (In fact the ring is heavy; it "wants to come as close to the ground, or to the center of the earth, as possible.) Both parts of the inextensible string, AX and BX, are stretched, and so the ring, sliding along the string, describes and ellipse with foci A and B. Obviously, the position of equilibrium is at the lowest point M of the ellipse where the tangent is horizintal.
    • George Pólya, Induction and Analogy in Mathematics, Vol. I of Mathematics and Plausible Reasoning (1954)
[edit]
Wikipedia
Wikipedia
Wikipedia has an article about:
Retrieved from "https://en.wikiquote.org/w/index.php?title=Ellipse&oldid=3056281"