If there is an integer such that
|
(1)
|
i.e., the congruence (1) has a solution, then is said to be a quadratic residue (mod
). Note that the trivial case
is generally excluded from lists of quadratic residues (e.g.,
Hardy and Wright 1979, p. 67) so that the number of quadratic residues (mod
) is taken to be one less than the number
of squares (mod
).
However, other sources include 0 as a quadratic residue.
If the congruence does not have a solution, then is said to be a quadratic
nonresidue (mod
).
Hardy and Wright (1979, pp. 67-68) use the shorthand notations
and
, to indicated that
is a quadratic residue or nonresidue, respectively.
In practice, it suffices to restrict the range to , where
is the floor function,
because of the symmetry
.
For example, ,
so 6 is a quadratic residue (mod 10). The entire set of quadratic residues (mod 10)
are given by 1, 4, 5, 6, and 9, since
|
(2)
| |
|
(3)
| |
|
(4)
|
making the numbers 2, 3, 7, and 8 the quadratic nonresidues (mod 10).
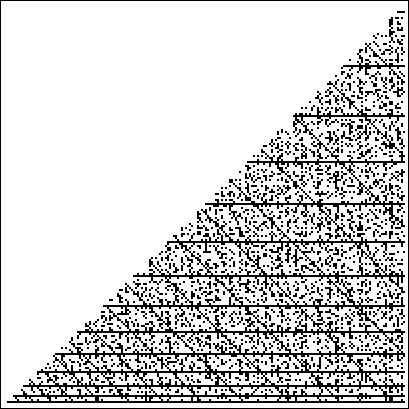
A list of quadratic residues for is given below (OEIS A046071),
with those numbers
not in the list being quadratic nonresidues of
.
| quadratic residues | |
| 1 | (none) |
| 2 | 1 |
| 3 | 1 |
| 4 | 1 |
| 5 | 1, 4 |
| 6 | 1, 3, 4 |
| 7 | 1, 2, 4 |
| 8 | 1, 4 |
| 9 | 1, 4, 7 |
| 10 | 1, 4, 5, 6, 9 |
| 11 | 1, 3, 4, 5, 9 |
| 12 | 1, 4, 9 |
| 13 | 1, 3, 4, 9, 10, 12 |
| 14 | 1, 2, 4, 7, 8, 9, 11 |
| 15 | 1, 4, 6, 9, 10 |
| 16 | 1, 4, 9 |
| 17 | 1, 2, 4, 8, 9, 13, 15, 16 |
| 18 | 1, 4, 7, 9, 10, 13, 16 |
| 19 | 1, 4, 5, 6, 7, 9, 11, 16, 17 |
| 20 | 1, 4, 5, 9, 16 |
The numbers of quadratic residues (mod ) for
, 2, ... are 0, 1, 1, 1, 2, 3, 3, 2, 3, 5, 5, 3, 6, 7, 5,
3, ... (OEIS A105612).
The largest quadratic residues for , 3, ... are 1, 1, 1, 4, 4, 4, 4, 7, 9, 9, 9, 12, 11, ...
(OEIS A047210).
Care must be taken when dealing with quadratic residues, as slightly different definitions are also apparently sometimes used. For example, Stangl (1996) adopts the apparently
nonstandard definition of quadratic residue as an integer satisfying
such that
and
is relatively prime to
.
This definition therefore excludes non-units (mod
). By this definition, the quadratic residues (mod
) for
, 2, ... are illustrated below (OEIS A096103,
the numbers of them are given by 0, 1, 1, 1, 2, 1, 3, 1, 3, 2, 5, 1, 6, ... (OEIS
A046073) and the number of squares
in
is related to the number
of quadratic residues in
by
|
(5)
|
for
and
an odd prime (Stangl 1996). (Note that both
and
are multiplicative
functions.)
| non-unit squares (mod | |
| 2 | 1 |
| 3 | 1 |
| 4 | 1 |
| 5 | 1, 4 |
| 6 | 1 |
| 7 | 1, 2, 4 |
| 8 | 1 |
| 9 | 1, 4, 7 |
Given an odd prime and an integer
, then the Legendre symbol
is given by
|
(6)
|
If
|
(7)
|
then
is a quadratic residue (+) or nonresidue (
). This can be seen since if
is a quadratic residue of
, then there exists a square
such that
, so
|
(8)
|
and
is congruent to 1 (mod
) by Fermat's little theorem.
Given
and
in the congruence
|
(9)
|
can be explicitly computed for
and
of certain special forms:
 |
(10)
|
For example, the first form can be used to find given the quadratic residues
, 3, 4, 5, and 9 (mod
, having
), whereas the second and third forms determine
given the quadratic residues
, 3, 4, 9, 10, and 12 (mod
, having
), and
, 3, 4, 7, 9, 10, 11, 12, 16, 21, 25, 26, 27, 28, 30, 33,
34, 36 (mod
,
having
).
More generally, let
be a quadratic residue modulo an odd prime
. Choose
such that the Legendre symbol
. Then defining
|
(11)
| |||
|
(12)
| |||
|
(13)
|
gives
|
(14)
| |||
|
(15)
|
and a solution to the quadratic congruence is
|
(16)
|
Schoof (1985) gives an algorithm for finding with running time
(Hardy et al. 1990). The congruence can solved
by the Wolfram Language command PowerMod[q,
1/2, p].
The following table gives the primes which have a given number
as a quadratic residue.
| primes | |
| 2 | |
| 3 | |
| 5 | |
| 6 |
Finding the continued fraction of a square root
and using the relationship
|
(17)
|
for the th
convergent
gives
|
(18)
|
Therefore,
is a quadratic residue of
. But since
,
is a quadratic residue, as must be
. But since
is a quadratic residue, so is
, and we see that
are all quadratic residues of
. This method is not guaranteed to produce all quadratic residues,
but can often produce several small ones in the case of large
, enabling
to be factored.