Replacing the logistic equation
|
(1)
|
with the quadratic recurrence equation
|
(2)
|
where
(sometimes also denoted
)
is a positive constant sometimes known as the "biotic potential" gives the so-called logistic
map. This quadratic map is capable of very complicated
behavior. While John von Neumann had suggested using the logistic map
as a random number generator in the late
1940s, it was not until work by W. Ricker in 1954 and detailed analytic studies
of logistic maps beginning in the 1950s with Paul Stein and Stanislaw Ulam that the
complicated properties of this type of map beyond simple oscillatory behavior were
widely noted (Wolfram 2002, pp. 918-919).
The first few iterations of the logistic map (2) give
|
(3)
| |||
|
(4)
| |||
|
(5)
|
where
is the initial value, plotted above through five iterations (with increasing iteration
number indicated by colors; 1 is red, 2 is yellow, 3 is green, 4 is blue, and 5 is
violet) for various values of
.
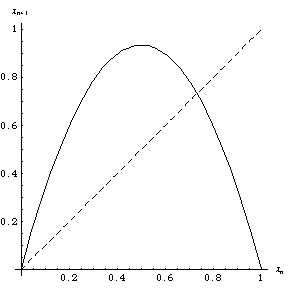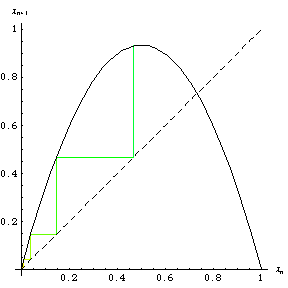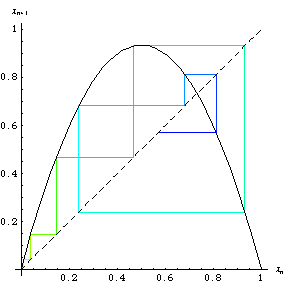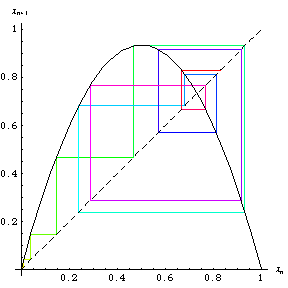
The logistic map computed using a graphical procedure (Tabor 1989, p. 217) is known as a web diagram. A web
diagram showing the first hundred or so iterations of this procedure and initial value
appears on the cover of Packel (1996; left
figure) and is animated in the right figure above.
In general, this recurrence equation cannot be solved in closed form. Wolfram (2002, p. 1098) has postulated that any exact solution must be of the form
|
(6)
|
where
is some function and
is its inverse function. M. Trott (pers.
comm.) has shown that smooth solutions cannot exist for generic values of
, with the possible exception of
even and nonzero. The only exact solutions known are for r=-2, r=2
and r=4, summarized in the table below
(Wolfram 2002, p. 1098),
and R. Germundsson (pers. comm., Apr. 25, 2002) has proved that no other
solutions of this form are possible.
| solution | ||
| 2 | ||
| 4 |
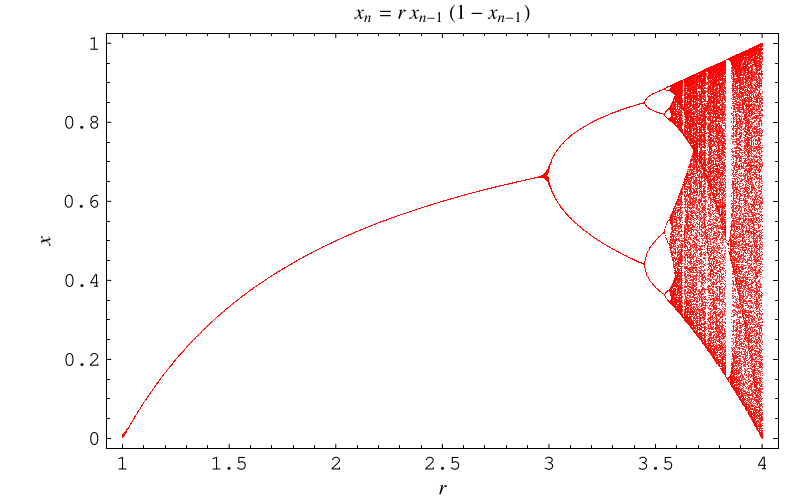
The illustration above shows a bifurcation diagram of the logistic map obtained by plotting as a function of
a series of values for
obtained by starting with a random value
, iterating many times, and discarding the first points corresponding
to values before the iterates converge to the attractor. In other words, the set
of fixed points of
corresponding to a given value of
are plotted for values of
increasing to the right.
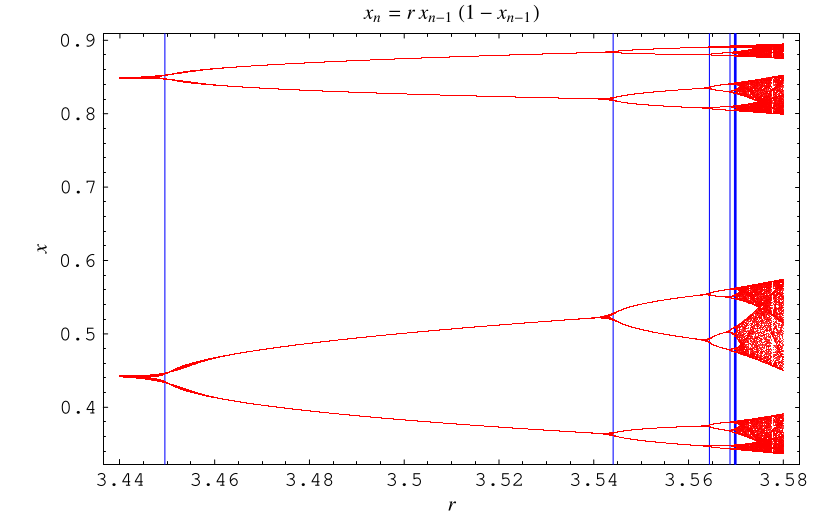
An enlargement of the previous diagram around is illustrated above, with value of
at which a
-cycle first appears indicated by blue lines.
In order to study the fixed points of the logistic map, let an initial point lie in the interval
. Now find appropriate conditions on
which keep points in the interval. The maximum value
can take is found from
|
(7)
|
so the largest value of
occurs for
.
Plugging this in,
.
Therefore, to keep the map in the desired region, we must
have
.
The Jacobian is
|
(8)
|
and the map is stable at a point if
.
Now find the fixed points of the map, which occur when .
For convenience, drop the
subscript on
|
(9)
| |
|
(10)
|
so the fixed points are and
.
An interesting thing happens if a value of greater than 3 is chosen. The map becomes unstable and we
get a pitchfork bifurcation with two stable
orbits of period two corresponding to the two stable fixed
points of
.
The fixed points of order two must satisfy
, so
|
(11)
| |||
|
(12)
| |||
|
(13)
| |||
|
(14)
|
For convenience, drop the
subscripts and rewrite
|
(15)
| |
|
(16)
| |
|
(17)
|
Notice that we have found the first-order fixed points as well, since two iterations of a first-order fixed point produce a trivial second-order fixed point. The true 2-cycles are given by solutions to the quadratic part
|
(18)
| |||
|
(19)
| |||
|
(20)
| |||
|
(21)
|
These solutions are only real for , so this is where the 2-cycle
begins. Note that the 2-cycle can also be found by computing the discriminant
of
|
(22)
|
which is
|
(23)
|
When this equals 0, two roots coincide, so is the onset of period doubling. For
, the solutions
are given by (0, 0,
) and (
,
,
3), so the first bifurcation occurs at
.
In general, the set of
equations which can be solved to give the onset of an arbitrary
-cycle (Saha and Strogatz 1995) is
|
(24)
|
The first
of these give
,
, ...,
, and the last uses the fact that the onset of period
occurs by a fold
bifurcation, so the
th
derivative is 1. For small
, these can be solved exactly, but the complexity rapidly increases
with
.
Now look for the onset of the 3-cycle. To eliminate the 1-cycles, consider
|
(25)
|
This gives
|
(26)
|
The roots of this equation are all imaginary for
less than some cutoff
,
at which point two of them convert to real roots.
The value of
can be found by computing the discriminant of (26),
|
(27)
|
When the discriminant is zero, two roots coincide. This happens at
|
(28)
|
(OEIS A086178) as first shown by Myrberg in 1958, so the 3-cycle starts at . Saha and Strogatz (1995) give a simplified algebraic treatment
for the 3-cycle which involves solving
|
(29)
|
together with three other simultaneous equations, where
|
(30)
| |||
|
(31)
| |||
|
(32)
|
Further simplifications still are provided in Bechhoeffer (1996) and Gordon (1996), but neither of these techniques generalizes easily to higher cycles. Bechhoeffer (1996) expresses the three additional equations as
|
(33)
| |||
|
(34)
| |||
|
(35)
|
giving
|
(36)
|
This has the positive solution found previously, .
Gordon (1996) derives not only the value for the onset of the 3-cycle, but also an upper bound
for the
-values
supporting stable period-3 orbits. This value is related to the unique positive root
of the cubic
equation
|
(37)
|
by
|
(38)
|
which is the unique positive root of the sextic polynomial
|
(39)
| |||
|
(40)
|
(OEIS A086179). For ,
|
(41)
| |||
|
(42)
| |||
|
(43)
|
Solving the resulting cubic equation using computer algebra gives
|
(44)
|
and ,
,
given by
|
(45)
|
giving numerical roots
|
(46)
| |||
|
(47)
| |||
|
(48)
| |||
|
(49)
| |||
|
(50)
| |||
|
(51)
| |||
|
(52)
| |||
|
(53)
| |||
|
(54)
| |||
|
(55)
|
where
is the silver constant.
To find the onset of the 4-cycle, eliminate the 2- and 1-cycles by considering
|
(56)
|
This gives a 12th-order polynomial in . The value of
can be found by computing the discriminant
of this polynomial,
 |
(57)
|
whose only real positive roots are
|
(58)
| |||
|
(59)
| |||
|
(60)
| |||
|
(61)
|
The 4-cycle therefore starts at
|
(62)
|
(OEIS A086180).
The onset of 5-cycles can be found analytically, and gives a 22nd-order polynomial in whose real positive roots are
(OEIS A118452),
3.90557..., and 3.99026....
The onset of 6-cycles can be found analytically, and gives a 40th-order polynomial in whose real positive roots are
(OEIS A118453),
3.93751..., 3.97776..., and 3.99758....
The onset of 7-cycles can be found analytically, and gives a 114th-order polynomial in whose real positive roots are
(OEIS A118746),
3.77413..., 3.88602..., 3.92218..., 3.95102..., 3.96897..., 3.98474..., 3.99453...,
and 3.99939....
The onset of 8-cycles can be found analytically as the polynomial root of the 8th-order polynomial
|
(63)
| |||
|
(64)
|
(OEIS A086181; Bailey 1993; Bailey and Broadhurst 2000; Borwein and Bailey 2003, pp. 51-52).
The onset of 16-cycles at (OEIS A091517)
was originally found using an integer relation
calculation which determined that
is the root of a 120th-degree integer
polynomial with coefficients that decrease monotonically from
to 1 (Bailey and Broadhurst 2000; Borwein and Bailey
2003, pp. 52-53). This result was subsequently verified exactly using computer
algebra (Borwein and Bailey 2003, p. 53; Kotsireas and Karamanos 2004), and
is an algebraic number of degree 240.
The following table summarizes the values at which the
-cycle first appears. For
, 2, ..., these have algebraic degrees 1, 1, 2, 2, 22, 40,
114, 12, ... (OEIS A118454).
| OEIS | value | ||
| 1 | 1 | 1 | |
| 2 | 1 | 3 | |
| 3 | 2 | A086178 | 3.82842712... |
| 4 | 2 | A086180 | 3.44948974... |
| 5 | 22 | A118452 | 3.73817237... |
| 6 | 40 | A118453 | 3.62655316... |
| 7 | 114 | A118746 | 3.70164076... |
| 8 | 12 | A086181 | 3.54409035... |
| 9 | |||
| 10 | |||
| 16 | 240 | A091517 | 3.56440726... |
The algebraic orders of the values of (i.e., the onset of the
-cycle) for
, 2, ... are therefore given by 1, 2, 12, 240, ... (OEIS
A087046). A table of the cycle
type and value of
at which the cycle
appears is given below.
| cycle ( | OEIS | ||
| 1 | 2 | 3 | |
| 2 | 4 | 3.449490 | A086180 |
| 3 | 8 | 3.544090 | A086181 |
| 4 | 16 | 3.564407 | A091517 |
| 5 | 32 | 3.568750 | |
| 6 | 64 | 3.56969 | |
| 7 | 128 | 3.56989 | |
| 8 | 256 | 3.569934 | |
| 9 | 512 | 3.569943 | |
| 10 | 1024 | 3.5699451 | |
| 11 | 2048 | 3.569945557 | |
| accumulation point | 3.569945672 | A098587 |
For additional values, see Rasband (1990, p. 23). Note that the table in Tabor (1989, p. 222) is incorrect, as is the entry in Lauwerier (1991). The period doubling bifurcations
come faster and faster (8, 16, 32, ...), then suddenly break off. Beyond a certain
point known as the accumulation point, periodicity
gives way to chaos, as illustrated below. In the middle
of the complexity, a window suddenly appears with a regular period like 3 or 7 as
a result of mode locking. The period-3 bifurcation
occurs at
,
and period doublings then begin again with cycles of 6, 12, ... and 7, 14, 28, ..., and then once
again break off to chaos. However, note that considerable
structure can be found within this chaos (Mayoral and Robledo 2005ab).
It is relatively easy to show that the logistic map is chaotic on an invariant Cantor set for (Devaney 1989, pp. 31-50; Gulik
1992, pp. 112-126; Holmgren 1996, pp. 69-85), but in fact, it is also chaotic for all
(Robinson 1995, pp. 33-37; Kraft 1999).
The logistic map has correlation exponent (Grassberger and Procaccia
1983), capacity dimension 0.538 (Grassberger
1981), and information dimension 0.5170976
(Grassberger and Procaccia 1983).
The logistic map can be used to generate random numbers (Umeno 1998; Andrecut 1998; Gonzáles and Pino 1999, 2000; Gonzáles et al. 2001ab; Wong et al. 2001, Trott 2004, p. 105).